Why staying centred matters: Lessons from Gothic cathedrals to modern crane outrigger stability

FIGURE 2: Pinnacles of the Reims Cathedral, completed c. 1275.

The builders of the cathedrals in the Middle Ages used the structures they were building as full-scale models in an organic process where the response of the structure to the applied loads served as signals to the builders that adjustments to the design were required. “What the devil does this have to do with operating cranes?” you are likely asking. Bear with me.

FIGURE 1: Lines of thrust in a Gothic Cathedral.

The most identifiable characteristic of the Gothic cathedrals is arguably the pointed arch (Figure 1). These arches function by resisting the gravity forces with horizontal forces called thrusts. The function of the structure is (as with a crane’s outrigger) to safely get those loads into the ground. The combination of these thrusts with the weights of the stone blocks results in a line of thrusts as the load follows the path to ground.

Lines of thrust

The builders noticed that as the structures got bigger, cracks were developing in the walls supporting the arches. The design of the cathedrals was governed by the paths that these lines of thrust took. Ideally you want the lines to point straight down to the ground. However, the horizontal thrusts were hindering this. Large stone walls, called buttresses, were built to resist these thrusts. And as the buildings got bigger, horizontal arms called flying buttresses, were added to support the upper walls.

They discovered that if the lines of thrust travelled much beyond the centre of the stone mass that those cracks would appear. Their solution was to rotate the lines of thrust to the vertical by adding weights, called pinnacles, to the tops of the buttresses. That is the reason that they added those wonderful statues to the tops of the structures (Figure 2).

In the mid-19th century, engineers began quantifying the behaviour of masonry structures.1 By the early 1900s the concept of “the middle third” was well in use in the design of masonry structures.

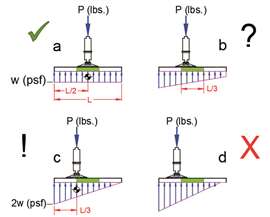FIGURE 3: Outrigger supported by crane mat upon the ground.

Let’s apply this concept to the problem of getting the significant loads from the outrigger floats into the supporting ground. The structure of interest is the crane mat. Its purpose is to distribute the sizeable point load from the outrigger float along the surface of the earth. (We will assume that the mat is sufficiently rigid, so flexure is not an issue. If you want to explore the subject of the support of cranes upon the ground in more depth, you should secure yourself a copy of David Duerr’s Mobile Crane Support Handbook, Second Edition, 2019).

The “standard” case (Case a in Figure 3) is where the crane mat is centred below the outrigger float. When you ask an engineer to calculate the ground bearing pressure below your outrigger, this is the assumed condition. The pressure beneath the crane mat is simply the total load divided by the area of the crane mat (again, here we are disregarding flexure and are assuming the mat to be 100% effective). So GBP, w = P/A where w = ground bearing pressure (psf), P is the outrigger load (lbs.), A is the area of the crane mat (ft2).

If you place the mat somewhat off centre, the maximum ground bearing pressure will increase beneath the mat as shown in Figure 3b. Note that the average ground bearing pressure beneath the mat is still the same. Depending on conditions, this may (or may not) be acceptable.

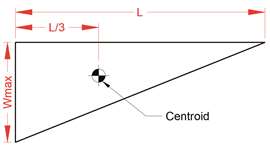FIGURE 4: The Triangle.

Once you push the outrigger out above the middle third of the mat (Figure 3c), the load at the mat’s far end is zero. This is because the centroid of the triangle (that’s where its centre of gravity is located) is one third of the leg length (Figure 4).

Because the total load must still be resisted, and recalling that the area of a triangle is ½ the base x height, the maximum pressure beneath the mat is now twice what it was in case a with the outrigger centred. The entire surface of the mat is involved in resisting the load, but the pressure upon the ground is uneven. This is unlikely to be acceptable, and a serious evaluation should be performed.

Revised standard

Last February, The American Society of Mechanical Engineers (ASME) issued a revision of their standard P30-1, Planning for Load Handling Activities. The new edition, ASME P30.1-2024 includes a new Appendix, Nonmandatory Appendix D, that includes a good treatment of many important topics related to the support of cranes upon the ground.

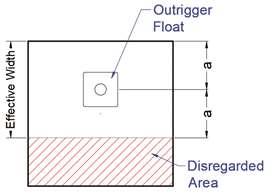FIGURE 5: Effective mat size.

Apropos of our discussion here, they provide guidance for eccentric loading of outrigger pads (sec. D-8.4). They suggest that if it is not possible to centre the outrigger float upon the mat, then consider an effective area such that the width and length dimensions are centred about the float (See Figure 5). The area outside the area of symmetry is to be disregarded. In the sketch that they present in the Appendix, the general case where the float has been offset in two dimensions is illustrated. In my discussion above, we have been assuming a case where the float has been slid in a single dimension to one third of the mat’s width. Thus, the effective area for this case would be two thirds of the mats area. The average ground pressure would then be 3/2 = 1.5 times the pressure of the case with the float centred. But because the distribution is uneven, the peak pressure (as I show above) is increased by a factor of two.

Going further, once the outrigger is placed beyond the middle third (Figure 3d) the entire mat is no longer involved in resisting the load and the pressure upon the ground is uneven and increases greatly. Don’t do this. Stay centred!

Geoffrey H Goldberg

Geoffrey H Goldberg is a senior project engineer at a major national crane operator. Previously, he spent 20 years designing bridges in the “City of Bridges” - Pittsburgh, PA.

He is the Author of Bridges: A Postcard History and several articles that have appeared in American Cranes and Transport (ACT).